Understanding Integers: The Key to Unlocking Mathematical Concepts
The Complete GuideWhen talking about numbers, the word “integer” may come up. But what exactly is an integer and why is it important in mathematics? Break it down in a simple, meaningful way, even if it’s not about math.
What is an integer?
An integer is an integer. This means no fractions, no decimals and something fun like a round slice and a half measure. Any number can be written without a fraction or decimal part. Here’s the fun part – integers can be positive, negative, or zero.Think of it this way: Integers are like the building blocks of the numerical universe. It’s simple, it’s simple, it’s versatile. Whether you’re counting apples, calculating numbers, or working out your temperature in degrees Celsius, all numbers are included.
Integer Example
Let’s look at some examples to make this clearer. Positive integers include numbers like 1, 5, and 97. These are the numbers you know best, the numbers you use every day. However, negative integers are numbers like -5, -97, or -3043. These are the same as positive integers, but with a minus sign in front, indicating that they are less than zero. So zero is just a special kind of integer – neither positive nor negative, just zero.So if we display some integers, it might look like this: -5, -1, 0, 1, 5, 8, 97, 3043. Pretty cool, right?
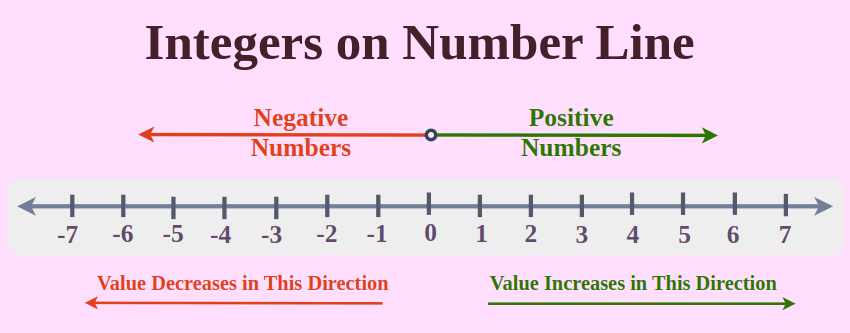
What is not an integer?
Now that we know what integers are, it’s important to know what zeros are. Numbers like 1.5, -0.67, or 3/4 are not integers. what Because fractions or decimals can be converted into something other than whole numbers. For example, 3.14 (which can be understood as close to pi) is not an integer. Not because it’s a negative 0.1 at the end of 5,643.1.So, whenever you see a number that contains a decimal or a fraction, you know it’s not an integer.Arrays of integersIn math, we like to group things, especially integers. The set of all integers is denoted by the symbol Z. Don’t ask what Z is—you, we mathematicians love it. The set of integers contains all the numbers we have discussed and extends to infinity on both sides. It usually goes down through bad numbers, from zero to good numbers.The set of integers is like this: Z = {…, -3, -2, -1, 0, 1, 2, 3, …}. There are three dots on each side and so on – there’s no end to the integers!
Integers in Equations
When you see a math equation, integers appear as numbers we don’t know yet. These unknown integers are represent by letters such as p, q, r, and s. It’s like the algebra says, “We don’t know what this number is yet, but we know it’s an integer.“So, if you see an equation with p or s, you can bet those letters are for the integer we’re trying to figure out.
Calculation of Integers
Now here’s the better part. A set of integers that mathematicians call “uncountable”. This is a nice way to say that even though there are infinitely many integers, we can list them in a way that covers them all. For example, you can write all the integers as {…, -3, -2, -1, 0, 1, 2, 3, …} and this list goes on forever and not skipping years.This concept is important because it helps mathematicians understand the size of infinity. Even if the set of integers is infinite, it is always counted, which is the real meaning.Multiply Integers and Natural NumbersHere’s a fun fact: you can combine any integer with a natural number (any integer from 1). For example, you can combine 1 with -1, 2 with 0, 3 with 1, and so on. This duality shows that the set of integers is the same “big” as the set of natural numbers, even though both are infinite.
Cardinality of Sets
of IntegersCardinality is a term that refers to the size of a set, and in mathematics it is a common practice to compare the size of infinite sets. The set of integers has the same cardinality as the set of natural numbers, which means they have the same size. But here’s the catch: combinations like real numbers (which include fractions and decimals) and complex numbers (which include imaginary numbers) are bigger! These sets have a greater cardinality than the set of integers, indicating that some infinities are greater than others. Crazy, right?
What is the significance of an integer?
You may be asking yourself, “What is the significance of an integer?” Well, integers are the backbone of algebra and algebra. These are the numbers we use to count, measure and calculate everything from simple math problems to complex equations in engineering and science. Without whole numbers, our number system would not work like this. They are like the solid blocks that make up the entire structure of mathematics.
How to use integers in the real world Everyday
integers are everywhere. When you check the temperature and it’s -5 degrees outside, it’s an integer. When you calculate your bank balance and find you’re $100 in the red, that’s also a negative number. Even if you count steps on your fitness tracker, it’s a nice whole number.Integers are also important in computer programming, engineering and understanding sports results. They help navigate the world with clear, consistent, and reliable figures.
Read More: Unlock Your Future: RRB NTPC Recruitment 2024 Notification Expected Soon
Conclusion
The Power of IntegersIntegers seem simple and intuitive, but they have a larger purpose. They help us count, measure and understand the world in a simple and intuitive way. For better, worse, or worse, integers surround us and shape the way we think about numbers and mathematics.Understanding.







